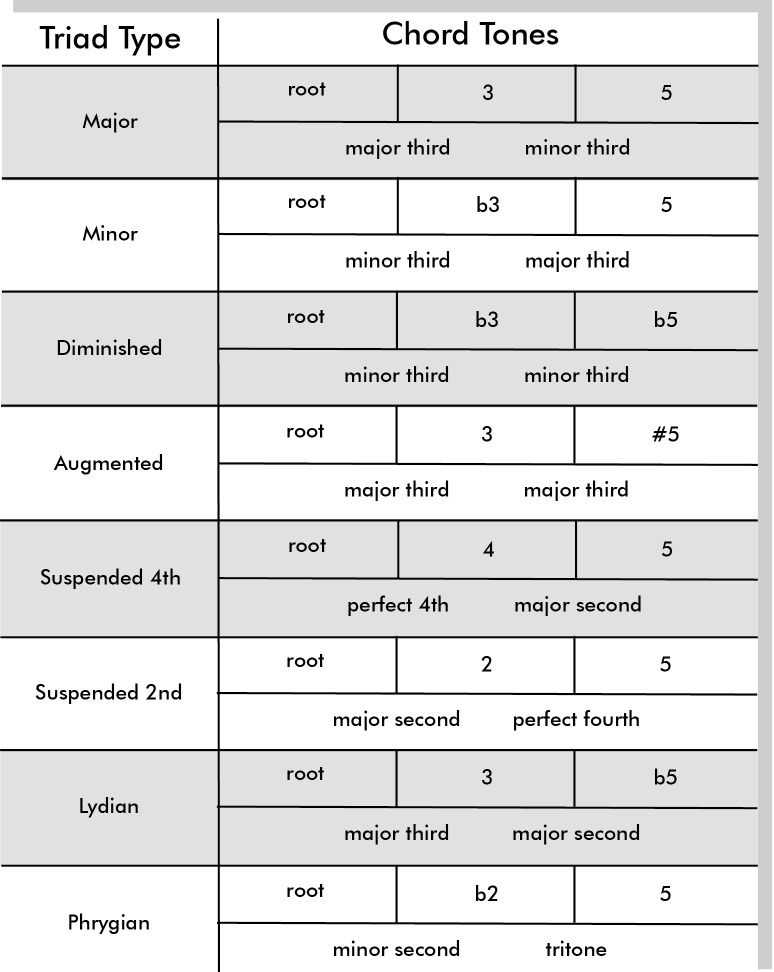
TRIADS & EXTENSIONS
- Rodrigo Moreira
- Mar 23, 2023
- 11 min read
Updated: 2 days ago
TABLE OF CONTENTS (Click to jump) |
Greetings, everyone!
To fully grasp the content of my article on “Triads & Extensions”, it is essential that you have a solid understanding of extensions, the distinction between available tensions and avoided notes, and how to use them for the various types of chords. I strongly recommend that you read all of the previous topics in chronological order before diving into this article. To access each topic, simply click on the links below:
Simples Intervals -> Compound Intervals -> Triads -> Drop-2 Chords -> Drop-3 Chords -> Shell Chords & Extensions -> Triads & Extensions -> Chord Melody -> Guitar Arpeggios -> Guitar Scales -> Major Keys -> Minor Keys -> Harmonic Minor Keys -> Melodic Minor Keys -> Greek Modes -> Chord Progressions
If you found my content informative and valuable, leave a comment with your feedback at the bottom of this page. Your input is very important to me, and I appreciate any insights you can provide. Thank you for your support and let’s get to work!
TRIADS & EXTENSIONS
In this article, we'll be combining the triad types we covered in the "Triads" article with the extensions from "Shell Chords & Extensions." Specifically, we'll explore the available tensions for major, minor, diminished, augmented, suspended 4th, and Lydian triads. While we have previously discussed two other types of triads, namely the Phrygian triad and suspended 2nd triad, I won't look deeply into them here. Their application depends on your knowledge of scales and Greek modes, so we'll revisit them later when relevant.
DOMINANT FUNCTION
To help you understand the concept of the dominant function, let's first break down the structure of two different 7th chords: Cmaj7 and C7. The Cmaj7 chord consists of the notes C (root), E (major 3rd), G (perfect 5th), and B (major 7th). In contrast, the C7 chord contains C (root), E (major 3rd), G (perfect 5th), and Bb (minor 7th). The only distinction between the major 7th chord and the dominant 7th chord lies in their seventh degree. A major seventh chord comprises a major triad and a major seventh, while a dominant 7th chord consists of a major triad and a minor seventh.
To avoid confusion, I will differentiate major triads by their function before discussing their available tensions. When I refer to "major triads with dominant function," I am talking about major triads that can replace any dominant chord. On the other hand, when I use the terms "major triad" or "major triad without dominant function," I am referring to major triads that can substitute any major 7th chord. This distinction will help us understand the specific applications of each type of major triad in our chord progressions.
Then, the major triad without dominant function shares the same available tensions as the major 7th chord, namely the 9th, #9th, #11th, and 13th. Conversely, the major triad with dominant function has the same available tensions as the dominant 7th chord, which includes the b9th, 9th, #9th, #11th, b13th, and 13th.
All chords we label as "dominant" share the same application, including the Cmaj7(#5) and C7(#5) chords. The only distinction between these two is their seventh. The Cmaj7(#5) is made up of an augmented triad with a major seventh, while the C7(#5) consists of an augmented triad with a minor seventh. Similarly, the available tensions for the augmented triad without dominant function are the same as those for the major 7(#5), which are the 9th and #11th. On the other hand, the available tensions for the augmented triad with dominant function are the same as those for the dominant 7(#5), which include the b9th, 9th, #9th, and #11th.
Lastly, the Cmaj7(b5) and C7(b5) chords can be separated by their functions as well. The Cmaj7(b5) comprises a Lydian triad with a major seventh, whereas the C7(b5) consists of a Lydian triad with a minor seventh. The available tensions for the Lydian triad without dominant function are the same as those for the major 7(b5), which include the 9th, b13th, and 13th. Meanwhile, the Lydian triad with dominant function has the same available tensions as the dominant 7(b5), including the b9th, 9th, #9th, b13th, and 13th.
As we progress into topics such as “Major and Minor Keys”, we will dive into the concept of “Harmonic Functions”, which consists of Tonic, Dominant, and Subdominant Functions. In music, every chord plays a unique role in creating tension and resolution. Dominant chords generate tension that subsequently resolves into tonic chords, while subdominant creates movement. In the following weeks, we will unravel these concepts in greater detail. In the meantime, focus on mastering the triads with their extensions and applying them to as many songs as you can!
I’m adding here the Table of Tensions and the Table of Triads again for reference.
TABLE OF TENSIONS

TABLE OF TRIADS
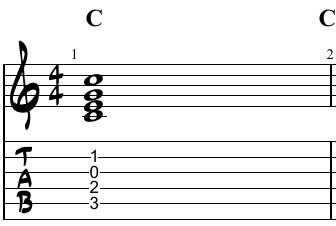
The following examples demonstrate triads in root-position and their inversions, including their extensions. The chords will always be in a four-note voicing format and played on the middle strings.
MAJOR TRIAD
C major on the middle strings [R 3 5 R]
C major on the middle strings w/ add9 [R 3 5 9]
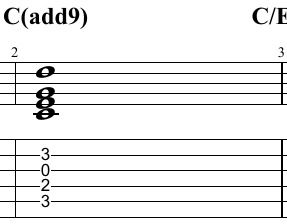
C major on the middle strings w/ add#9 [R 3 5 #9]
C major on the middle strings w/ add#11 [3 5 R #11]
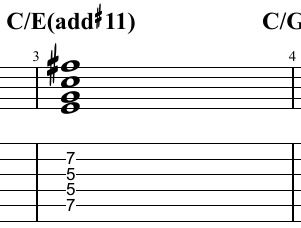
C major on the middle strings w/ add13 [5 R 3 13]
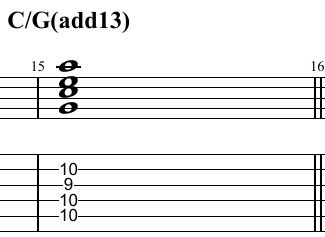
The Ionian mode (first degree of the major scale) generates the tensions 9th and 13th.
The Lydian mode (fourth degree of the major scale) generates the tensions 9th, #11th, and 13th.
*The Lydian #2 mode (sixth degree of the harmonic minor scale) generates the tensions #9th, #11th, and 13th.
MINOR TRIAD
C minor on the middle strings [R b3 5 R]
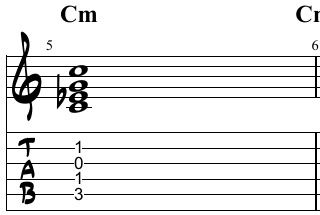
C minor on the middle strings w/ add9 [R b3 5 9]

C minor on the middle strings w/ add11 [b3 5 R 11]

C minor on the middle strings w/ add#11 [b3 5 R #11]
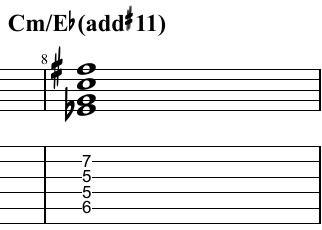
C minor on the middle strings w/ add13 [5 R b3 13]
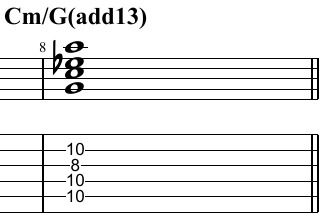
The Aeolian mode (sixth degree of the major scale) generates the tensions 9th and 11th.
The Phrygian mode (third degree of the major scale) generates the tension 11th.
The Dorian mode (second degree of the major scale) generates the tensions 9th, 11th, and 13th.
**The Dorian #4 mode (fourth degree of the harmonic minor scale) generates the tensions 9th, #11th, and 13th.
MAJOR TRIAD WITH DOMINANT FUNCTION
C major on the middle strings [R 3 5 R]

C major on the middle strings w/ addb9 [R 3 5 b9]

C major on the middle strings w/ add9 [R 3 5 9]

C major on the middle strings w/ add#9 [R 3 5 #9]

C major on the middle strings w/ add#11 [3 5 R #11]

C major on the middle strings w/ addb13 [5 R 3 b13]

C major on the middle strings w/ add13 [5 R 3 13]

The Mixolydian mode (fifth degree of the major scale) generates the tensions 9th and 13th.
The Lydian b7 mode AKA Mixolydian #4 mode (fourth degree of the melodic minor scale) generates the tensions 9th, #11th, and 13th.
The Mixolydian b6 mode (fifth degree of the melodic minor scale) generates the tensions 9th and b13th.
The Mixolydian b2b6 mode (fifth degree of the harmonic minor scale) generates the tensions b9th and b13th.
The Half-whole diminished scale AKA Dominant diminished scale generates the tensions b9th, #9th, #11th, and 13th.
The fourth degree remains an avoided note for dominant chords, as it is for all major chords. Although the dissonance between the third and fourth degrees may not be problematic, it does alter the chord's character.
DIMINISHED TRIAD
C diminished on the middle strings [R b3 b5 R]

C diminished on the middle strings w/ add9 [R b3 b5 9]
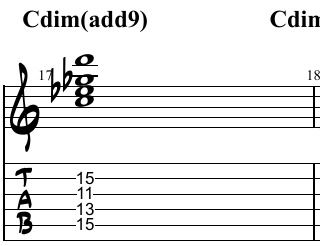
C diminished on the middle strings w/ add11 [b3 b5 R 11]
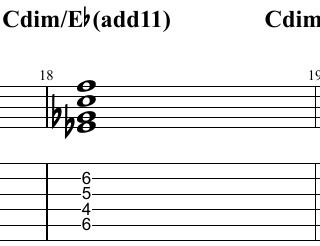
C diminished on the middle strings w/ addb13 [b5 R b3 b13]
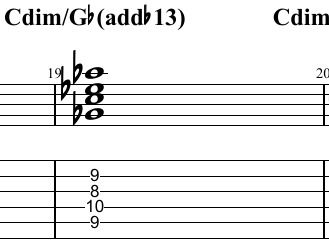
C diminished on the middle strings w/ addb15 [b5 R b3 b15]

The Symmetrical Diminished scale generates the tensions 9th, 11th, b13th, and b15th.
****Unlike most scales and modes, the symmetrical diminished scale is an octatonic scale that features an additional note, Cb (key of C), as its final degree. This Cb is classified as an available tension and is referred to as "addb15" due to its compound interval. While some musicians might label this note as a major seventh, choosing to call it B instead of Cb, this practice leads to confusion. This is because the diminished seventh chord already incorporates another seventh, namely, the Bbb (diminished seventh). For this reason, it is recommended to name it Cb.
SUSPENDED 4TH TRIAD
C suspended 4th on the middle strings [R 4 5 R]

C suspended 4th on the middle strings w/ addb9 [R 4 5 b9]

C suspended 4th on the middle strings w/ add9 [R 4 5 9]

C suspended 4th on the middle strings w/ addb17 [R 4 5 b17]

C suspended 4th on the middle strings w/ add17 [4 5 R 17]
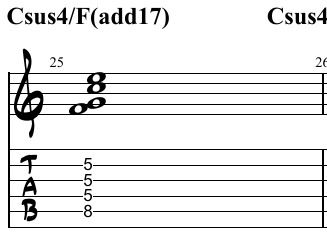
C suspended 4th on the middle strings w/ addb13 [5 R 4 b13]
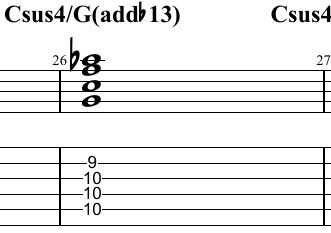
C suspended 4th on the middle strings w/ add13 [5 R 4 13]
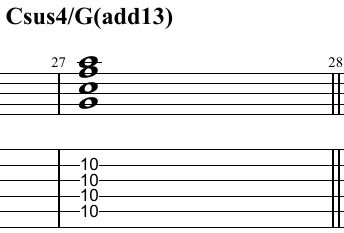
The Mixolydian mode (fifth degree of the major scale) generates the tensions 9th, 13th, and 17th.
The Dorian b2 mode (second degree of the melodic minor scale) generates the tensions b9th, 13th, and b17th.
The Phrygian mode (third degree of the major scale) generates the tensions b9th, b13th, and b17th.
The 17th is actually a major third while the b17th is a minor third. When it comes to 7sus4 chords, the 3rd is no longer considered a chord tone, but instead becomes an available tension. You may be wondering if adding the third will cause the chord to sound dissonant or change its quality. The answer is that it depends on how you add the tension. If the third is added above the fourth, a seventh interval will be created between the two, which is different than if it were done the opposite way. If the third is the lowest note of the chord and the fourth is above it, a minor 9th interval will result and create unwanted dissonance. Additionally, the further apart the third and fourth are from each other, the less tension there will be between them. This is why we refer to it as the 17th instead of the 10th, even though they are the same notes.
AUGMENTED TRIAD
C augmented on the middle strings [R 3 #5 R]

C augmented on the middle strings w/ add9 [R 3 #5 9]
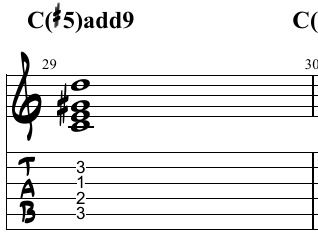

The Lydian #5 mode AKA Lydian augmented (third degree of the melodic minor scale) generates the tensions 9th and b13th.
LYDIAN TRIAD
C Lydian triad on the middle strings [R 3 b5 R]

C Lydian triad on the middle strings w/ add9 [R 3 b5 9]
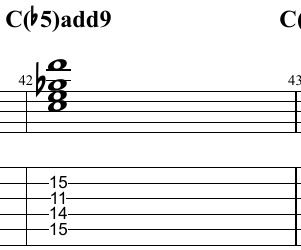
C Lydian triad on the middle strings w/ addb13 [b5 R 3 b13]

C Lydian triad on the middle strings w/ add13 [b5 R 3 13]
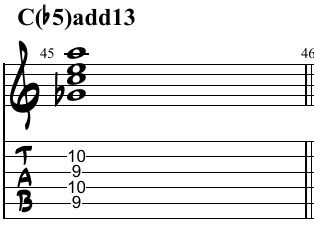
The Lydian #5 mode AKA Lydian augmented (third degree of the melodic minor scale) generates the tensions 9th and b13th.
******The Lydian mode (fourth degree of the major scale) generates the tensions 9th and 13th.
AUGMENTED TRIAD WITH DOMINANT FUNCTION
C augmented on the middle strings [R 3 #5 R]

C augmented on the middle strings w/ addb9 [R 3 #5 b9]
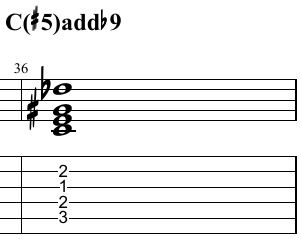
C augmented on the middle strings w/ add9 [R 3 #5 9]



The Whole-tone scale generates the tensions 9th and #11th.
The Altered scale AKA Super Locrian and Altered dominant scale generates the tensions b9th, #9th, and #11th.
LYDIAN TRIAD WITH DOMINANT FUNCTION
C Lydian triad on the middle strings [R 3 b5 R]

C Lydian triad on the middle strings w/ addb9 [R 3 b5 b9]

C Lydian triad on the middle strings w/ add9 [R 3 b5 9]

C Lydian triad on the middle strings w/ add#9 [R 3 b5 #9]
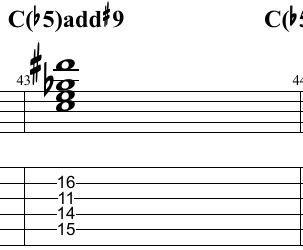
C Lydian triad on the middle strings w/ addb13 [b5 R 3 b13]

C Lydian triad on the middle strings w/ add13 [b5 R 3 13]

The Whole-tone scale generates the tensions 9th and b13th.
The Altered scale AKA Super Locrian and Altered dominant scale (seventh degree of the melodic minor scale) generates the tensions b9th, #9th, and b13th.
The Half-whole diminished scale AKA Dominant diminished scale generates the tensions b9th, #9th, and 13th.
The Lydian b7 mode AKA Mixolydian #4 mode (fourth degree of the melodic minor scale) generates the tensions 9th and 13th.
HOW PRACTICE TRIADS & EXTENSIONS?
Unlike the preceding articles, I’m going to bring a new idea on how to practice these chords. This will require some familiarity with scales and modes, and if you are unfamiliar with these concepts, please hold off until I publish supplementary information on the blog in the next few weeks. This does not exclude the exercises we have been practicing on chords since we first began with triads and 7th-chords. Exploring different ways of playing songs and practicing chords both horizontally and vertically will help you develop fluency on your instrument over time.
1) To harmonize a scale or mode, we can combine its triad and its inversions with their corresponding extensions. For instance, the following image demonstrates the mapping of C Lydian scale horizontally on the high e string (1st string).
[C Lydian mode on the high e string]

Locate the C major triad and its inversions such that all the chord tones (C, E, and G) are present on the top string.
[C Lydian mode on the high e string harmonized with C major triads]
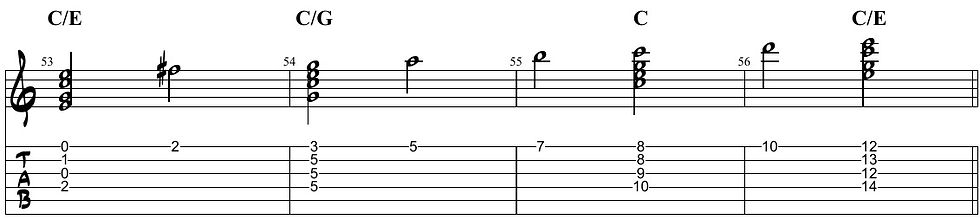
You may have observed that in all of the selected voicings, both the highest and lowest notes of each chord are identical. Therefore, we can modify the top note of each chord without compromising its quality. The next step is to include the remaining notes by altering the top note of each chord and ultimately reach the triad's extensions.
[C Lydian mode on the high e string harmonized with C major triads and its extensions]
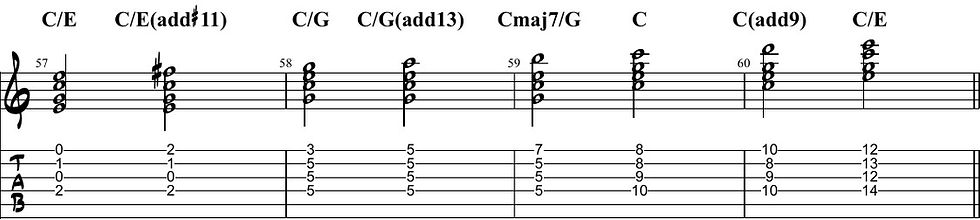
In the second measure, the C/E(add#11) is equivalent to the C/E chord from the first bar, with the top note altered to F#. Similarly, the C/G(add13) in the fourth measure is the same as the C/G chord from the third bar, but with the top note changed to A. In the fifth measure, we have the same triad as in the third measure, but with a major seventh added to the top. We could consider the major seventh an extension and call it the major 14th, but it would yield the same result as calling it a Cmaj7/G chord. On the seventh measure, the C(add9) is identical to the C chord from the sixth measure, except for the top note alteration. Lastly, the eighth measure features the octave of the first chord.
Apply the same process to all the scales and modes within your knowledge. After mapping everything out, try improvising your own solos by employing the same chord shapes. Observe how adding more notes to the melody creates a richer and more engaging sound, similar to how a pianist might play. This marks the start of comprehending how to create your own chord-melodies or arrangements, which we will dive into more deeply in the subsequent article.
2) To conclude, here are a few examples of how to implement Triads & Extensions to the first eight measures of "Autumn Leaves": (Cmin7 | F7 | Bbmaj7 | Ebmaj7 | Amin7(b5) | D7 | Gmin(maj7) | G7).
[Eight first measures of Autumn Leaves with Triads & Extensions Example 1]
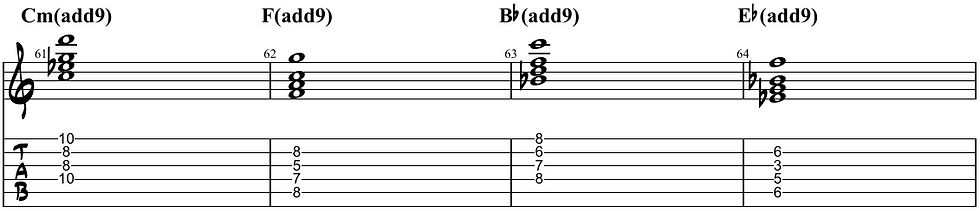
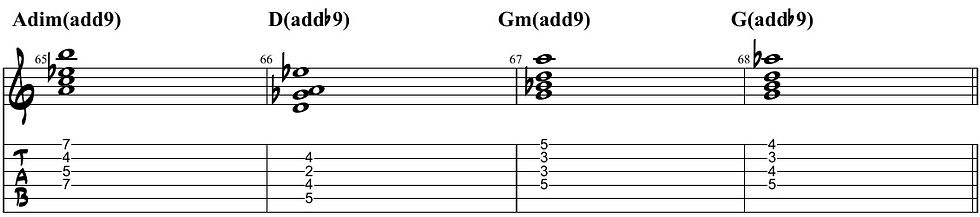
[Eight first measures of Autumn Leaves with Triads & Extensions Example 2]


[Eight first measures of Autumn Leaves with Triads & Extensions Example 3]
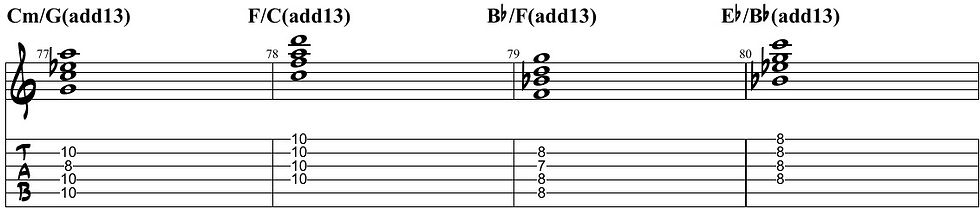
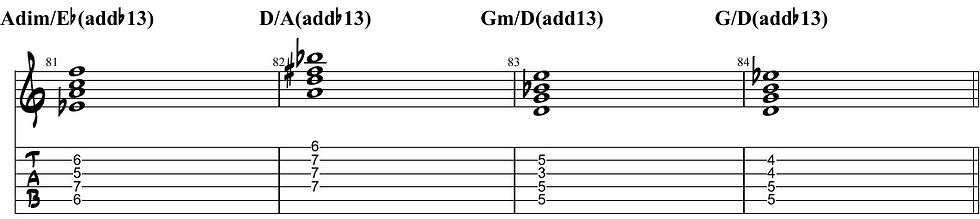
[Eight first measures of Autumn Leaves with Triads & Extensions Example 4]
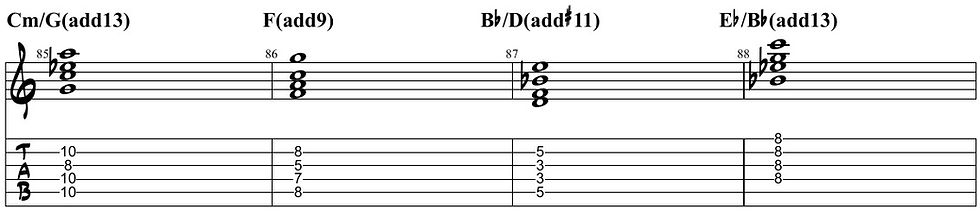

MORE IDEAS ON HOW TO PRACTICE TRIADS & EXTENSIONS?
*To access the full content of this website, click on "Music With No Shortcuts" in the top menu and read the complete description.





Comments